Casio ClassPad 330 V.3.04 - Handbuch (Seite 144)

20060301 20090601
2-4-16
Berechnungen mit vorinstallierten Funktionen
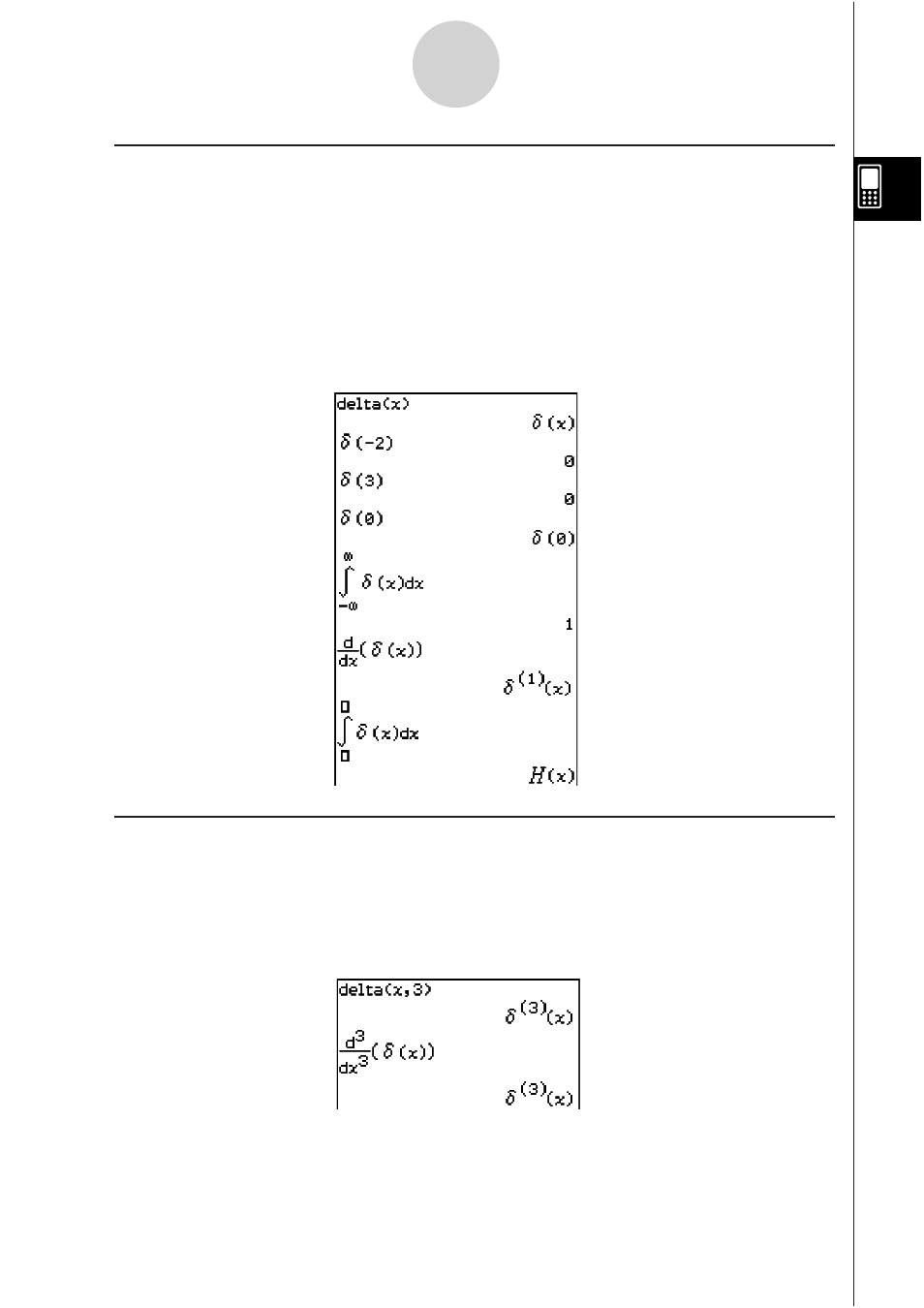
I Dirac-Delta-Distribution
„delta“ bezeichnet die Dirac-Delta-Distribution. Die Delta-Distribution evaluiert numerisch, wie
nachstehend dargestellt.
Nicht-numerische Terme, die in die Delta-Distribution übertragen werden, bleiben nicht-evaluiert.
Das Integral einer linearen Delta-Distribution ist eine Heaviside-Funktion.
Syntax: delta( x )
x : Variable oder Zahl
Beispiele:
I n -te Delta-Distribution
Die n -te Delta-Distribution ist das n-te Differential der Delta-Distribution.
Syntax: delta( x , n )
x : Variable oder Zahl
n : Anzahl von Differentialen
Beispiele:
0, x x 0
D(x) =
[
D( x ), x = 0
0, x x 0
D(x) =
[
D( x ), x = 0